Accelerating learning!

We have been doing fractions for the past two weeks... Mainly trying to get the students to understand what halves and quarters are, then moving them onto understanding that half a half is a quarter, so two quarters is the same as one half etc. Only two of the students in my class can independently find multiple parts of a whole, (e.g. 3/4, 5/6). The rest are still finding one of (e.g. 1/3, 1/4, 1/2)

In a one hour session today, I helped move three of my lower achieving students up in the strategy they use and how they express their thinking. 

We started with this... (which was irrelevant to our question, but that is besides the point.) The point is he couldn't explain verbally, visually or anyway how he got his answer. 

I explained to them how with fractions, the bottom number is how many groups you have. We practiced splitting numbers into groups and that the top number is how many groups you are looking at. For example, half of ten means you put ten into two groups (because the bottom number is 2) then look at how many are in one group (because the top number is a 1).

I started giving the students fraction word problems about 1/4, 1/2, 1/3 and they were getting it and could draw their thinking, and then talk about what they drew as a way of explaining how to get their answer. 

Our question here was -

'Dad has 20 cookies and gives 1/4 to each of his 3 children. The rest he gives to mum. How many cookies did mum get?

Notice how they have drawn four squares, (which shows me they understand that for quarters you need 4 groups), put dots in each box to represent the cookies (which shows me they understand how to share things equally), circled one box (which shows me they understand they are looking for ONE quarter, rather than two or three quarters) and written the number of cookies mum gets next to their drawing (which shows me they can find part of a whole).

How much learning can be seen in one snapshot of a students drawing is amazing isn't it...

They seemed to find quarters, halves and thirds easy by using this strategy, so I upped the anti and gave them fractions even the rest of the class hadn't seen yet. Sixths, sevenths, tenths etc, and asked them to find multiple parts.

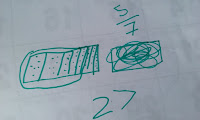
I gave the students variations of this word problem

'Miss Ashley has (X) number of lollies and gave (fraction) to the green table. How many lollies did she give them?'

Here is their thinking...

(K, C and L are the students initials, to protect their identity.)

 K - Miss Ashley has 20 lollies and gave 2/4 to the green table. How many lollies did she give them? Also seen here on the left side is the answer to 'Miss Ashley has 30 of lollies and gave 1/3 to the green table. How many lollies did she give them?'

 C -Miss Ashley has 35 lollies and gave 1/5 to the green table. How many lollies did she give them? 

 C - Miss Ashley has 35 lollies and gave 2/7 to the green table. How many lollies did she give them?

 K - Miss Ashley has 25 lollies and gave 2/5 to the green table. How many lollies did she give them?

 L - Miss Ashley has 35 lollies and gave 5/7 to the green table. How many lollies did she give them?

 L - Miss Ashley has 50 lollies and gave 9/10 to the green table. How many lollies did she give them?

L - Miss Ashley has 30 lollies and gave 8/10 to the green table. How many lollies did she give them?

 C - Miss Ashley has 30 lollies and gave 2/10 to the green table. How many lollies did she give them?

They went from barely knowing what halves and quarters were, to being able to find 9/10 and 5/7 of a  number. Incredible! I was so proud of them for stepping up such a HUGE amount during one hour of intensive teaching, and I could see they were buzzing and were proud of themselves as well. They were actually sad to pack up for morning tea!

Maths observation

Today Archana observed me taking maths with our class. 

I won't post the observation notes on here, but here is a summary.

Things I did well

"I liked the way you did number talks and warm up activity. You launched the problem and it was clearly worded for the students. You tried to use Talk Moves when students were sharing their mathematical thinking though the one that you used was mainly when students were repeating themselves."

Things to work on

Minimising talking during independent think time. 

(I can work on this by clearly stating my expectation of the noise level and behaviour before the students leave the mat, and praise them for working to my expectations throughout the think time). 

Leaving the students alone during independent think time - not prompting them.

(I can work on this by just looking at their work and not asking them questions about what they have written/drawn. If they ask me a question, I can tell them that we will talk about it later in whole class time). 

Having the WALT displayed and referring to it

(I can work on this by having the WALT written out on an A3 paper and putting it up everyday, rather than leaving the opportunity for me to forget to write it everyday. I also need to explain what it means and break down the words to ensure the students understand them.)

Pace of the lesson

(I can work on this by only doing 2 warm up questions with the squeezey boxes, and giving the students less time for the number talks. They have been doing this for a while now and don't need as much time as they used to.)

Modelling book

(I can work on this by having the question pre-written onto the modelling book and leaving it within my sights (not putting it to the side) so I remember to use it. I did take a clinic, but I did not the modelling book to record the groups thinking)

Encouraging metacognition

Everyday, I ask my students, "what did you learn today?"

And every time I ask them, they always have something to say. I accept (most) answers, as all learning matters. What they learn in maths and reading is important, yes, but so are developing social skills and their ability to think and talk about their own learning.

It is a class, syndicate and school wide goal to get our students to think more about their own thinking (metacognition) and to talk about their thinking. Simply asking them for one thing they learnt that day is a great way to get them to

• think about the new things they learnt that day
• to have their learning on their mind as they are walking out the door at the end of the day instead of what they did at lunchtime. 

This is Tuesday's response

Today I learnt... 23 March 2016 from Tamaki Primary on Vimeo.

You will notice a lot of them talked about their blogs, as we did a focus session on accessing their blogs and how to post for the first time (they are very new to their blogs).

I plan to show them this video, and talk about the benefits of talking about our learning, and different ways we can show what we have learnt.

Learning for/through/because of/by teaching.

This week has been a huge week.

I found myself waking up, and my first thought being 'I am so excited to hang out with my learners today'. I found myself coming home from school, and happily spending hours on planning, because I was so excited about their learning I couldn't stop myself.

I was working on this. 

Creating this site was so exciting for me. Is that nerdy to admit? I don't know, but I don't care.

We learnt how to make them on one of our digital immersion days, and have been taught little bits here and there of how to make them better. I was proud of my site and the planning I had done on it for my learners. I showed it to my mentor the next morning and she got excited too...

(and wanted to know how to make one too - teaching point for me!). She also left me an encouraging comment on the bottom of the site if you want to read it.

Then, at digital immersion today, we were shown how to insert invisible tables using HTML and how these make your site look better and also make it more adaptable on different devices, as photos, videos (etc.) are forced into place and can't shift around your web page. This is perfect for my learners who use netbooks, as their screen size is different to mine.

So then, I updated my site! It now has both WYSIWYG formatting and HTML formatting 

(I'm still learning! It won't be perfect just yet....)

My reflection on this site though is not about the formatting of my site, but how much learning has gone into it and come out of it.

Learning for teaching

I first learnt how to make a site to benefit my teaching skills and my learners.

Learning through teaching

I am continually learning what strategies, preferences, multimodal options (etc.) work best for my learners, when I am teaching them

Learning because of teaching

Teaching isn't static. It is always changing, and so my practice should be as well. As teachers we need to constantly be keeping up with 'the times' of effective/best practice, and using these digital tools is one of those things.

Learning by teaching

I am cementing my own learning by teaching these skills and knowledge to my mentor.

So many different types of learning and teaching, interlocked and intertwined, interdependent and interrelated. If that doesn't summarise being a teacher, I don't know what will.

Digital Immersion (day 7)

Today we had a field trip to Stonefields school for the Manaiakalanai School leaders study tour.
Their principal Sarah Martin did a seminar about collaboration and communication that I got a lot out of. As the principal, she obviously spends a lot of time with her staff, and with an ever-growing number of staff and having 11 new teachers this year, she used a lot of strategies to get everybody on board and on an equal playing field. Along with that huge number of staff comes with collaborative issues. How can people work together when they all are so different? How can we prevent elephants in the room becoming conflicts? How do we deal with conflict when it arrises?

One thing she talked about was having a shared vision of where you want to be. What she did with her staff, which I plan to now do with my students in our own way, was get them to draw where they think they are now in terms of their collaboration, and then where they want to be. This gave them a vision and gave the staff strategies for when there are elephants in the room.

So...
Food for thought...


Where do I think I am with my collaborative efforts?

The person on the left is me, with a strong connection to and from my mentor teacher. I recognise that I only have bonds with some of the other staff members of my school, and that there are some people I do not collaborate with.

Goal for the future?

Get to know everyone! I need to build relationships with those people that I may not necessarily see everyday - people in the junior syndicate and support staff in particular. This will build my collaborative community as I can then talk to these people about anything and give and receive support!

Learning to create or creating to learn?

This week we have started serious work using our netbooks and introduced our student blogs. 

At Digital Immersion (day 5)  we talked about the difference between learning to create, and creating to learn. And yes, there is a difference. 

Learning to create - where learning is the process and the 'creation' is the final outcome. 

Creating to learn - where creating is the process and learning is the final outcome.

Which is better? Is one better than the other? Why or why not?

That made me think about my learners and their blogs. This week, I have been trying to teach them about their netbooks. I felt they need to gain the knowledge and skills about how to use their netbooks before they can use them in-depth for learning. However, they actually are sort of picking most things up a long the way all by themselves. They may not know the names of the different parts of their netbooks, but they are figuring out how to use them...

So... am I teaching them to learn how to create, or teaching them to create to learn?

Which should I be doing? Why is that one better?

Or should I be doing a mixture of the two... Explicitly telling them things they probably won't discover on their own, but also letting them figure out for themselves what they can..

What do you think?

Digital Immersion (day 5)

Sourced from: https://commons.wikimedia.org/wiki/File:Logo_Google_Sites.png

Today we talked a lot about using Google Sites for effective practice, and how in creating your Site we are determining access to learning for students and their families.

We discussed the ten top principles for effective web design -

1. Usability and the utility
2. Obvious and self-explanatory
3. Remove all barriers - no access denied or sign up required
4. Focus users attention - visual cues, carefully chosen text
5. 3 Clicks ( or 1-2-3-done-steps)
6. Effective writing
7. Simplicity - Clean
8. Embrace White Space - not crowded
9. Conventions are our friends - consistent layout, icons, Home button etc
10. Test early, Test often.  Check using Incognito or Safari

We then critically analysed some sites provided to us, for their;

1. learner engagement
2. Whanau access (including language used)
3. use of multimodal learning

Our end goal is to create our own site where we can use the best of all we learnt. 

Just for today though, we discussed, critically analysed and sourced resources for our chosen current event.

Here is my slide from our slideshow -

We then will use this planning to create and share our current events site next week!

Digital Immersion (day 4)

Today we talked a lot about Google Sites, and touched on other background things such as writing HTML and embedding outsider gadgets.

As I use a ready-made syndicate site at school, and my PRT site was made from a template, I have not made a site from scratch before. It may seem a bit bland to make a 'sandpit' site, one designed purely for someone to play around it, for hours, but it afforded me a lot of learning opportunities.

I now know how to create a site, change all of its settings, layout and design features, turn particular features on/off, add content from many sources and then 'bling' it up (aka making it pretty).

It will be awesome to create on I can use for my learners, as I can design it in such a way that makes sense to myself and my learners.

I also learnt a little bit about how to read, write, edit and embed HTML code.

Here is a little Youtube explanation of what HTML is.

Note: this Youtube video is inserted into this blog post using HTML! 

We played around with inputting resources from different sources into a Google Site, and shared these with each other. 

Impromptu Observations

During my release time today, I was supposed to be completing our weekly plan for next week. However as I sat in the class sorting out what I needed etc, I became aware of an amazing lesson going on right before my eyes. I instantly started recording notes, unbeknown to the teacher (my mentor, Archana). Of course she figured out that I was observing her when I quickly went and took photos of the whiteboard, kept typing while she spoke and didn't appear to be doing any planning haha! But wow, the results were amazing!

The focus I chose was seeing how many times the teacher either used talk moves, or praised the students, and in what way. See for yourself... (hint: I have highlighted the key pieces.)

As you can see, there was soo much rich use of talk moves, maths language and positive behaviour reinforcement used by the teacher in this hour session. The more I analysed the results, the more it made me think about what kind of things I say to the students...

(I have taken the actual observation out of this post for privacy reasons, as it contains personal information about students including their names.)

I want to note that I don't want to replicate what my mentor teacher has done, as I am my own person and not a clone of her, however wonderful she may be. My point is, that maybe I need to watch what I say to the students, and how I am saying it. How can I get the same level of engagement and excitement about maths?  Do I refer to our class treaty that much? Do I build them up just for trying that much? Do I connect with them on a personal level, still in whole class situations? If not, how can I? How can I set such a tone for the love of learning?

Lecture #1 (740)

Today was our first lecture as part of the MDTA programme and I am feeling inspired!

I thought it would take me a long time to get back into the swing of learning 'lecture style' after not having been in lectures since October, but no way! 

First Rebecca talked us through the purpose of our paper (EDCURRIC740) and we discussed accelerating learning; the teachers role, the students role, 'the black box', effective teaching and critical thinking. These all related to our paper 740 but also our daily classroom teaching.

Next Nina Hood got us to do a good ole' pen and paper survey. When I do these, I tend to go with my first thought and not over-analyse the statements or why I am choosing what Im choosing. 

Here is mine -

Click here for some of the discussion points for each one.

If you made it till the end, congratulations! I hope you were inspired as I was to use technology in its best way, selecting the best tools for each purpose, and to not forget some students are going to prefer to keep the ole' pen and paper, and thats okay too!